Order Ideals on Lexicographic Direct Sum of Three Totally Ordered Abelian Groups

Dian Latifah, Sumanang Muhtar Gozali, Rizky Rosjanuardi, Imam Nugraha Albania

Abstract


Order ideals play an important role in the study of abstract algebra, especially in the study of ordered groups. In this paper, we focus on the study of order ideals in lexicographic direct sums of totally ordered Abelian groups. We begin by examining the order ideals in the group of integers , and the group of real numbers  It is shown that there are no non-trivial order ideals in both groups. Next, we revisit the order ideals in the lexicographic direct sum of two totally ordered Abelian groups,  The only non-trivial order ideal of  is  Furthermore, our study extends to the lexicographic direct sum of three totally ordered Abelian groups:  and  We investigate the non-trivial order ideals in these structures. It is stated that the non-trivial order ideals of  are only  and  Furthermore, the non-trivial order ideals of  are only  and .

Keywords: order ideal; lexicographic order; direct sum.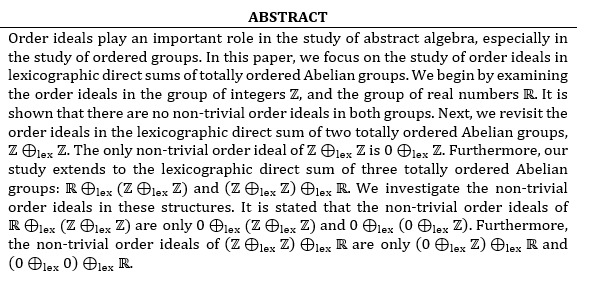


Keywords


order ideal; lexicographic order; direct sum

Full Text:

PDF

References


[1]

Hamouda, E. H, "Soft Ideals in Ordered Semigroups, " Revista De La Union

Matematica Argentina, pp. 85-94, 2016.

[2]

Muhiuddin, G, & Ahsan Mahboob, "Int-soft Ideals over the soft sets in ordered semigroups," AIMS Mathematics, pp. 2412-2423, 2020.

[3]

Ze Gu, Xiang-Yun Xie, & Jian Tang, "On C-ideals and the basis of an ordered semigroup," AIMS Mathematics, pp. 3783-3790, 2020.

[4]

Faiz Muhammad Khan, Tian-Chuan Sun, Asghar Khan, Muhammad Junaid, & Anwarud Din, "Intersectional soft gamma ideals of ordered gamma semigroups," AIMS Mathematics, pp. 7367-7385, 2021.

[5]

Yatir Halevi, & Assaf Hasson, "Strongly dependent ordered Abelian groups and Henselian field," Israel Journal of Mathematics, pp. 719-758, 2019.

[6]

Rosjanuardi, R, Yusnitha, I, & Gozali, S.M, "Representation of Cylically Ordered Semigroup," MATEC Web of Conferences 197, 01002, 2018.

[7]

Rosjanuardi, R, Yusnitha, I, & Gozali, S.M, "On linear cyclically ordered subgroups of Cylically Ordered groups," Journal Of Physics: Conference Series 1280 (2), 022044, 2019.

[8]

Rosjanuardi, R, Yusnitha, I, & Gozali, S.M, "Cylically Ordered group and C* dynamical system," Journal Of Physics: Conference Series 1280 (2), 022043, 2019.

[9]

Sumanang Muhtar Gozali; Rizky Rosjanuardi; I. N. Albania, "On c-convex subgroups of Z oplus Z as a cyclically ordered group," AIP Conference Proceedings 2614, 040053, 2023, https://doi.org/10.1063/5.0127481

[10]

Antonio Di Nola, Giacomo Lenzi, & Gaetano Vitale, "Expanding lattice ordered Abelian groups to Riesz spaces," Mathematica Slovaca, vol. 72, no. 1, 2022, pp. 1-10. https://doi.org/10.1515/ms-2022-0001

[11] Dale Rolfsen, "Low-dimensional topology and ordering groups,"Mathematica Slovaca, pp. 579-600, 2014.

[12] Kathryn Mann, & Cristobal Rivas, "Group ordering, dynamic, and rigidity," Ann. Inst. Fourier, pp. 1399-1445, 2018.

[13] Neset Ozkan Tan, "A lexicographical order induced by Schauder bases," Hacettepe Journal of Mathematics and Statistics, 511-515, 2021

[14] Frink, O, "Ideals in Partially Ordered Sets," The American Mathematical Monthly, pp. 223-234, 1954.

[15] Andrus, J. F., & Butson, A. T, "Ideals in Ordered Groups," The American Mathematical Monthly, 265-269, 1965.

[16] Adji, S., & Raeburn, I, "The Ideal Structure of Toeplitz Algebra," Integral Equation Operator Theory, 281-293, 2004.

[17] Adji, S., Raeburn, I., & Rosjanuardi, R, "Group Extensions and the Primitive Ideal Spaces of Toeplitz Algebras," Glasgow Mathematical Journal Trust, 81-92, 2007.

[18] Rosjanuardi, R, "Primitive Ideals of Toeplitz Algebra of Ordered Groups," J. Indones. Math. Soc. (MIHMI), 111-119, 2008.

[19] Rosjanuardi, R, "Characterization of Primitive Ideals of Toplitz Algebras of Quotients," J. Indones. Math. Soc. (MIHMI), 67-71, 2012.

[20] Rosjanuardi, R., & Itoh, T, "Characterization of Maximal Primitive Ideals of Toeplitz Algebras," Scientiae Mathematicae Japonicae Online, 393–397, 2010.

[21] Rosjanuardi, R., Gozali, S. M., & Albania, N. I, "c-Convex Subgroups of Finite Dimensional Cyclically Ordered Free Abelian Groups," International Journal of Mathematics and Computer Science, 37-45, 2023.

[22] Rosjanuardi, R., Gozali, S. M., & Yusnitha, I, "Twisted Toeplitz Algebras of Cyclically Ordered Groups," Journal of Physics: Conference Series, 2021.

[23] Davey, B. A., & Priestley, H. A. Introduction to Lattice and Order. Cambridge: Cambridge University Press, 2002.

[24] Goodearl, K. R. Partially Ordered Abelian Groups with Interpolation. Providence, Rhode Island: American Mathematical Society, 1986.




DOI: https://doi.org/10.18860/ca.v9i1.24116

Refbacks

  • There are currently no refbacks.


Copyright (c) 2024 Sumanang Muhtar Gozali, Dian Latifah, Rizky Rosjanuardi, Imam Nugraha Albania

Creative Commons License
This work is licensed under a Creative Commons Attribution-ShareAlike 4.0 International License.

Editorial Office
Mathematics Department,
Universitas Islam Negeri Maulana Malik Ibrahim Malang
Gajayana Street 50 Malang, East Java, Indonesia 65144
Faximile (+62) 341 558933
e-mail: cauchy@uin-malang.ac.id

Creative Commons License
CAUCHY: Jurnal Matematika Murni dan Aplikasi is licensed under a Creative Commons Attribution-ShareAlike 4.0 International License.